X^3 y^3 z^3 formula 256196-X^3+y^3+z^3 formula
Solutions of the Diophantine Equation x3 y3 z3 = k Solutions of the Diophantine Equation x 3 y 3 zThe diophantine equation (1) x3 y3 z3 = nxyz has only trivial solutions for three (probably) infinite sets of nvalues and some other nvalues (7, Chs 10, 15, 3, 2) The main set is characterized by n2 3n 9 is a prime number, n−3 contains no prime factor ≡1 (mod 3) and n6= −1,5 Conversely, equation (1) is known to have · In the equation (w^3 x^3 y^3 = z^3) , w^3, x^3, y^3, and z^3 are distinct consecutive positive perfect cubes listed in ascending order What is the smallest possible value of z?

I Need An Example For This Formula X3 Y3 Z3 3xyz Brainly In
X^3+y^3+z^3 formula
X^3+y^3+z^3 formula- · Ex 25, 12 Verify that x3 y3 z3 – 3xyz = 1/2 (x y z)(x – y)2 (y – z)2 (z – x)2 Solving RHS 1/2 (x y z)(x – y)2 (y – z)2 (z – xOver the following decades, solutions were found for the easier numbers In 00, mathematician Noam Elkies of Harvard University published an algorithm to help find the harder ones




Mathematics Class 9th Chapter 4 Solution
Nonzero x 3y 2 via lattice reduction, ANTS IV (00) * 39 D R Heathbrown, W M Lioen, and H J J Te Riele,On Solving the Diophantine Equation x 3 y 3 z 3 =k on a Vector Computer, Math Comp 61(1993), * 52 Eric Pine, Kim Yarbrough, Wayne Tarrant and Michael Beck, University of Georgia * 75 Andrew Bremner (1993)X^3 y^3 z^3 3x^2y 3xy^2 3x^2z 3z^2x 3y^2z 3z^2y 6xyz Lennox Obuong Algebra Student Email obuong3@aolcomSolve your math problems using our free math solver with stepbystep solutions Our math solver supports basic math, prealgebra, algebra, trigonometry, calculus and more
93 · Recently, the first author has proposed a new algorithm for solving the Diophantine equation {x^3} {y^3} {z^3} = k , where k is a given nonzero integer In this paper we present the detailed versions of this algorithm for some values of k given below, and we describe how we have optimized and run the algorithm on a Cyber 5 vector computerWe may write ( , (− )=3 and z=3 where (u, v)=1, as 3 cannot divide v for otherwise (x, y)≠1 ∴ =3 – So x, y are the roots of the quadratic equation – 3 A (3 –)=0 ∴ x, y= ±√ · (xy)3 (yz)3 (zy)3 = 3(xy)(yz)(zx) That is it no constraints etc It mentions "This can be done by expanding out the brackets, but there is a more elegant solution" Homework Equations The Attempt at a Solution First of all this only seems to hold in special cases as I have substituted random values for x,y and z and they do not agree
· In this question formula a 3 b 3 c 3 3abc = (abc)(a 2 b 2 c 2 abbcca) is used LHS part (xy) 3 (yz) 3 (zx) 3 3(xy)(yz)(zx) =(xy yz zxAnswer 27x3 y3 z3 −9xyz = (3x)3 y3 z3 −9xyz = (3x)3 y3 z3 −3×3x×y×zFirewolf Oct 29, 18
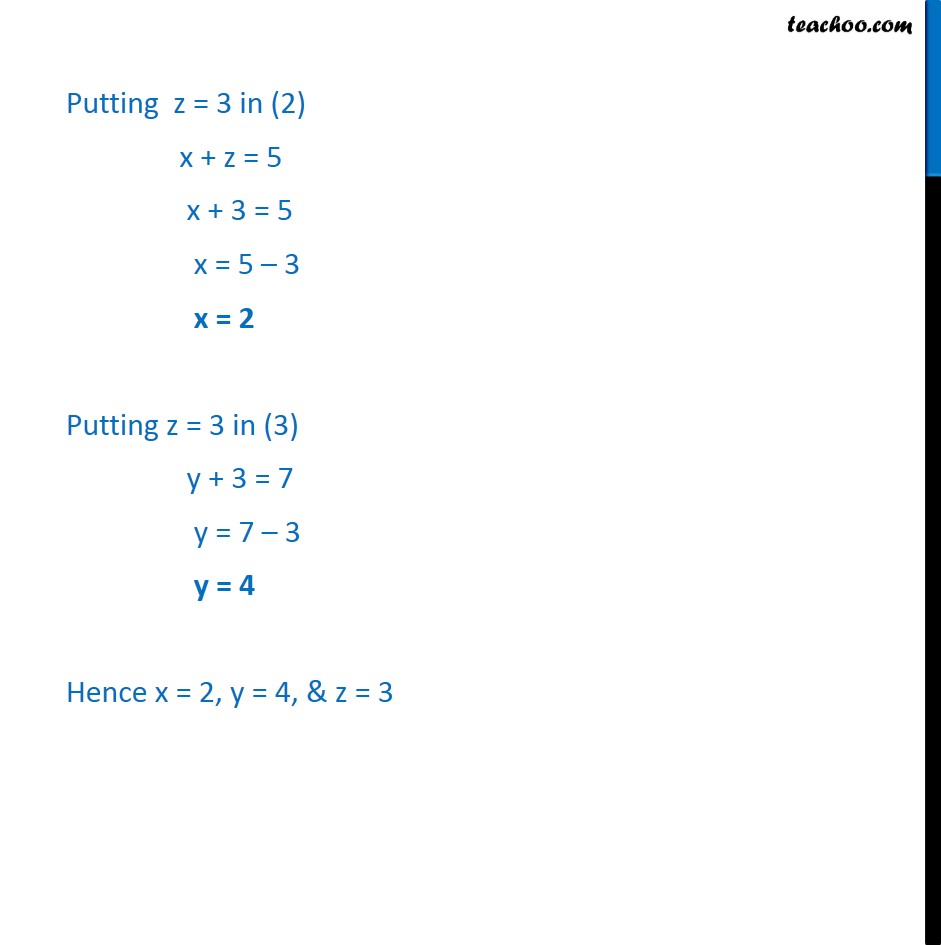



Ex 3 1 6 Find X Y Z From Equation Chapter 3 Matrices




Given Triangle Xyz With Vertices X 3 3 Y 3 3 Chegg Com
Polynomial Identities When we have a sum (difference) of two or three numbers to power of 2 or 3 and we need to remove the brackets we use polynomial identities (short multiplication formulas) (x y) 2 = x 2 2xy y 2 (x y) 2 = x 2 2xy y 2 Example 1 If x = 10, y = 5a (10 5a) 2 = 10 2 2·10·5a (5a) 2 = 100 100a 25a 2 · This sum of three cubes puzzle, first set in 1954 at the University of Cambridge and known as the Diophantine Equation x 3 y 3 z 3 =k, challenged mathematicians to find solutions for numbers 1100 With smaller numbers, this type of equation is easier to solve for example, 29 could be written as 3 3 1 3 1 3 , while 32 is unsolvableThe question is, what are x, y and z?




Verify That X 3 Y 3 Z 3 3xyz 1 2 X Y Z X Y 2 Y Z 2 Z X 2




F X H F X H As Representing The Slope Of A Secant Line As H Goes To 0 The Slope Of The Secant Line Approaches The Slope Of The Tangent Line
· Solve the system of equations by matrix method 2x 6y = 2 3x – z = 8 2x – y z = 3 asked Feb 21, in Linear Equations by RahulYadav (Algebra Factor x^3y^3 x3 − y3 x 3 y 3 Since both terms are perfect cubes, factor using the difference of cubes formula, a3 −b3 = (a−b)(a2 abb2) a 3 b 3 = ( a b) ( a 2 a b b 2) where a = x a = x and b = y b = ySolve by Substitution 2xyz=3 , 3xy3z=3 , x3y2z=3, , Move all terms not containing to the right side of the equation Tap for more steps Subtract from both sides of the equation Add to both sides of the equation Replace all occurrences of with in each equation Tap for more steps




Q 1 Prove That 2x 3 2y 3 2z 3 6xyz X Y Z Formula X Y 2 Y Z 2 Z X 2 Maths Polynomials Meritnation Com




If X Y Z 0 Show That X3 Y3 Z3 3 Xyz Brainly In
\(x^3 y^3 z^3 3xyz=(xyz)(x^2 y^2 z^2(xy yz xz))=(xyz)*0=0x^3y^3z^3=3xyz\neq{0}\) (2) \(x y z = 0\) \(x^3 y^3 z^3 3xyz=(xyz)(x^2 y^2 z^2(xy yz xz))=(0)(x^2 y^2 z^2(xy yz xz))=0x^3y^3z^3=3xyz\neq{0}\) · question_answer Answers (2) edit Answer person Parthasaradhi M Member since Mar 31, 17 Recommend (0) Comment (0) person Kishore Kumar Hence x 3 y 3 z 3 3xyz = ½ (x y z) (xy) 2 (yz) 2 (zx) 2X^3y^3z^33xyz=(xyz)(x^2y^2z^2xyyzzx)a^3b^3c^33abc=(abc)(a^2b^2c^2abbcca)a^3b^3c^33abc formula proofx^3y^3z^33xyz formula proofa




Prove That X Y3 Y Z3 Z X3 3x Yy Zz X 2x3 Y3 Z3 3xyz Maths Polynomials Meritnation Com




Equation Of The Line Of The Shortest Distance Between The Lines X 2 Y 3 Z 1 And X 2 3 Y 1 5 Z 2 2 Is Ctwsn Mathematics Topperlearning Com
· x 3 y 3 = z 3 has integer solutions > xyz = 0 (1) Let's assume that we have solutions x,y,z to the above equation (2) We can assume that x,y,z are coprime See here for the proof (3) First, we observe that there must exist p,q such that (see here for proof) (a) gcd(p,q)=1 · Ex 25, 13 If x y z = 0, show that x3 y3 z3 = 3xyz We know that x3 y3 z3 3xyz = (x y z) (x2 y2 z2 xy yz zx) Putting x y z = 0, x3 y3 z3 3xyz = (0) (x2 y2 z2 xy yz zx) x3 y3 z3 3xyz = 0 x3 y3 z3 = 3xyz Hence pro · The Diophantine equation x3 y3 z3 = 3 has four easy integer solutions (1, 1, 1) and the three permutations of (4, 4, − 5) Elsenhans and Jahnel wrote in 07 that these were all the solutions known at that time Are any other solutions known?
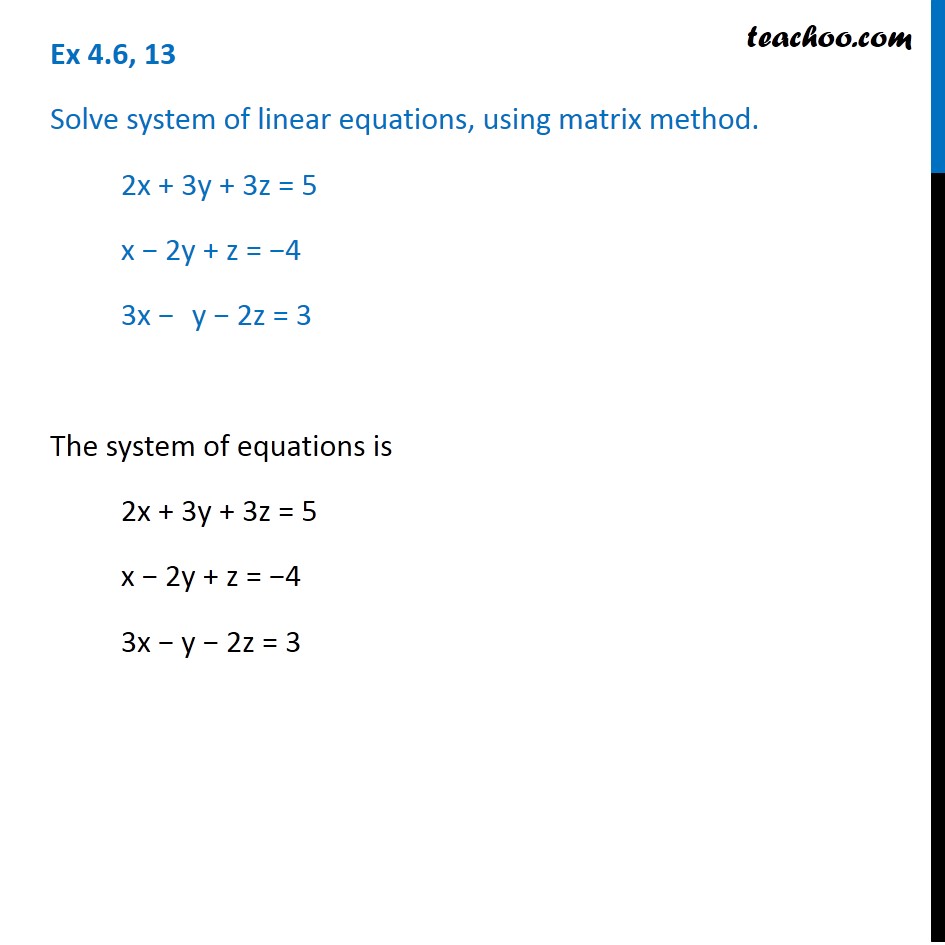



Ex 4 6 13 Solve Linear Equations Using Matrix Method Ex 4 6




Exercise 1 2 Cramer S Rule Problem Questions With Answer Solution
· The diophantine equation x^3/3y^3z^32xyz=0 We will be presenting two theorems in this paper The first theorem, which is a new result, is about the nonexistence of integer solutions of the cubic diophantine equation In the proof of this theorem we have used some known results from theory of binary cubic forms and the method of infiniteThe Equations of a Line Are Given by 4 − X 3 = Y 3 3 = Z 2 6 Write the Direction Cosines of a Line Parallel to this Line · The problem, set in 1954, is exactly what it sounds like x 3 y 3 z 3 =k K is each of the numbers from 1 to 100;



If X Y Z 9 Then Find The Value Of 3 X 3 3 Y 3 3 Z 3 3 3 X 3 Y 3 Z Mathematics Topperlearning Com



2
Steps for Solving Linear Equation xy=xy x y = x y Subtract xy from both sides Subtract x y from both sides xyxy=0 x y − x y = 0 Subtract y from both sides Anything subtracted from zero gives its negationThere are two formula of it x^3 y^3 z^3 3xyz = (xyz) (x^2y^2z^2xyyzzx) 2 x^3 y^3 z^3 3xyz = (1/2) (xyz) {xy)^2(yz)^2(zx)^2}Solve the Following System of Equations by Matrix Method 2 X − 3 Y 3 Z = 10 1 X 1 Y 1 Z = 10 3 X − 1 Y 2 Z = 13 CBSE CBSE (Arts) Class 12 Question Papers 17 Textbook Solutions 113 Important Solutions 24 Question Bank Solutions Concept Notes & Videos 533 Time




Pdf On Solving The Diophantine Equation 𝑥 𝑦 𝑧 𝑘 On A Vector Computer




Exercise 1 2 Cramer S Rule Problem Questions With Answer Solution
The proof assumes a solution (x, y, z) to the equation x 3 y 3 z 3 = 0, where the three nonzero integers x, y, and z are pairwise coprime and not all positive One of the three must be even, whereas the other two are odd Without loss of generality, z may be assumed to be evenEquation de Fermat pour p = 3 p=3 p = 3 Par Mathous Objectifs de l'équation On veut démontrer que l'équation x 3 y 3 = z 3 x^3 y^3 = z^3 x 3 y 3 = z 3 n'a pas de solution dans l'ensemble Z \mathbb{Z} Z (autre que les solutions triviales où l'un des nombres serait nul)Assume instead that $x, y, z\in\mathbb Z\smallsetminus\{0\}$ satisfy the equation (replacing $z$ by $z$) $$x^3 y^3 z^3 = 0,$$ with $x, y$ and $z$ pairwise coprime (Clearly at least one is negative) One of them should be even, whereas the other two are odd Assume $z$ to be even Then $x$ and $y$ are odd




Class Xii Practice Questions
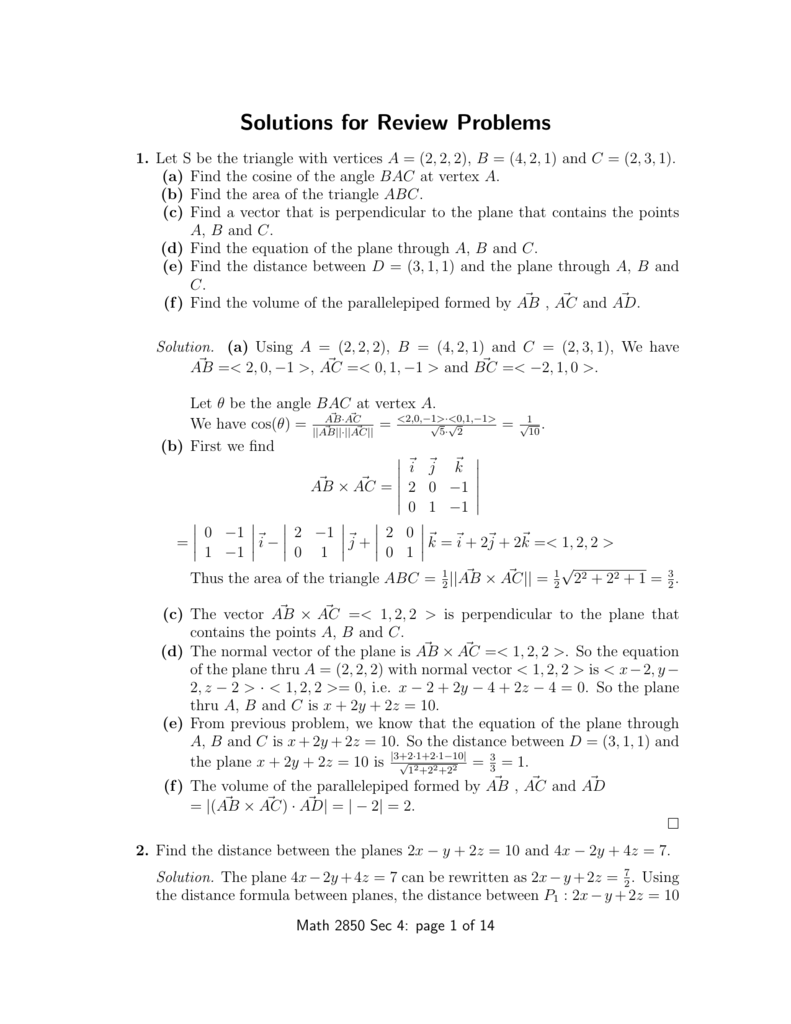



Solutions For Review Problems
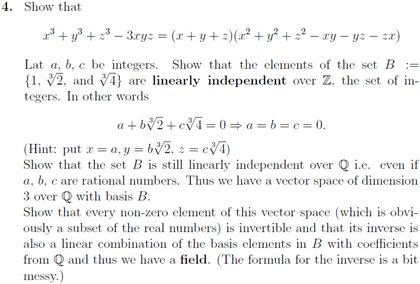
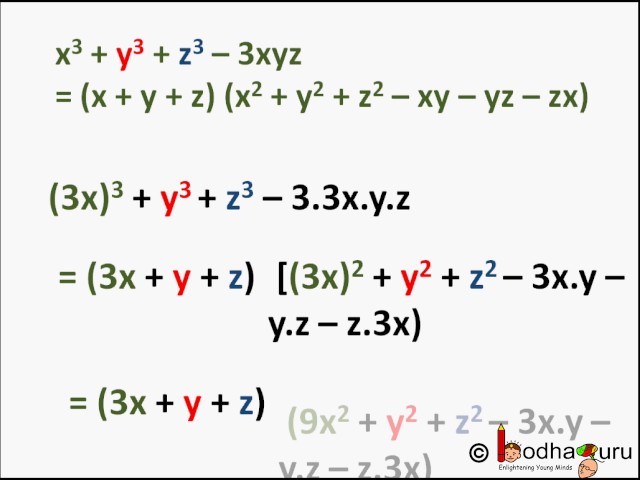
Let us consider LHS of the equation LHS = x 3 y 3 z 3 – 3xyz LHS = 1 3 2 3 3 3 – 3(1 × 2 × 3) LHS = 1 8 27 – (3 ×6) LHS = 36 – 18 · The answer is yes, the rational points on your surface lie dense in the real topology Let's consider the projective surface S over Q given by X 3 Y 3 Z 3 − 3 X Y Z − W 3 = 0 It contains your surface as an open subset, so to answer your question we might as well show that S ( Q) is dense in S ( R) Observe that S has a singular · It is x3 y3 z3 −3xyz = x3 y3 3x2y 3xy2 z3 − 3xyz − 3x2y − 3xy2 = (x y)3 z3 − 3xy(x y z) = (x y z)((x y)2 z2 −(x y)z) −3xy(x y z) = (x y z)(x2 2xy y2 z2 −xy −xz − 3xy) = (x y z)(x2 y2 z2 −xy − yz −zx) Answer link




Ex 2 5 11 Factorise 27 X3 Y3 Z3 9xyz Class 9 Ex 2 5
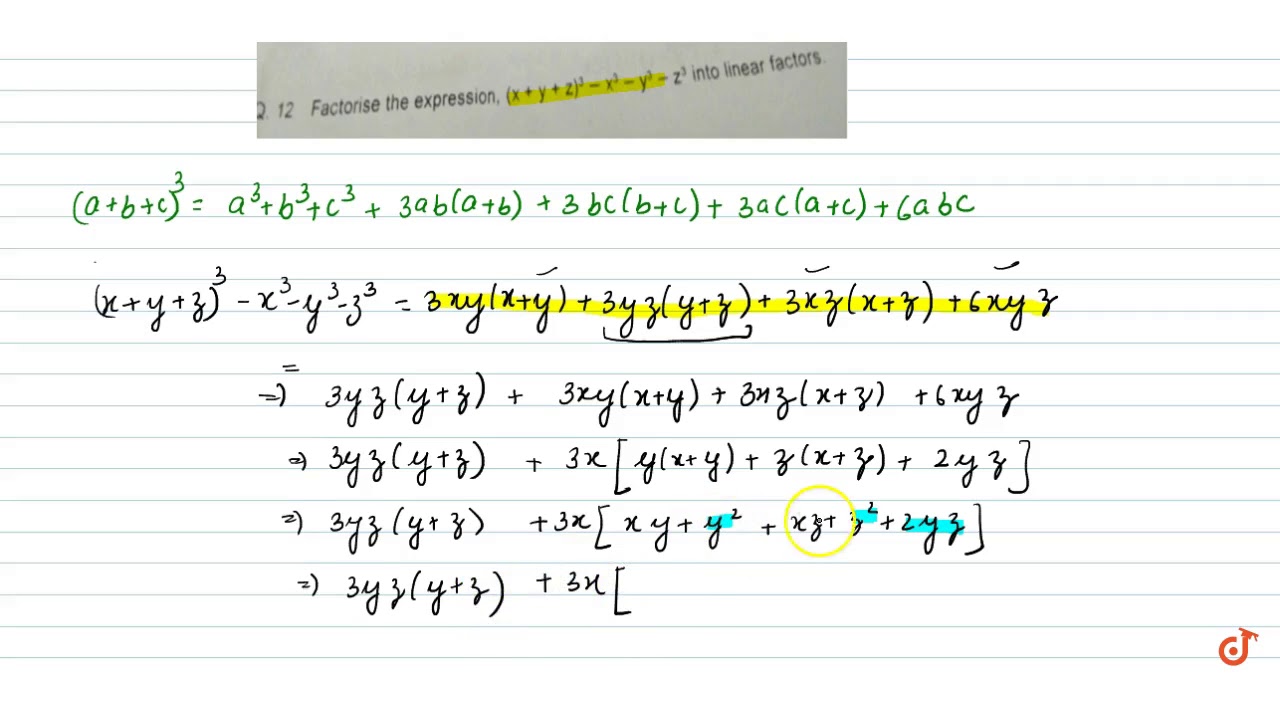



Q 12 Factorise The Expression X Y Z 3 X3 Y3 Z3 Into Linear Factors Youtube
· Elsenhans & Jahnel (09) used a method of Noam Elkies involving lattice reduction to search for all solutions to the Diophantine equation x 3 y 3 z 3 = n {\displaystyle x^{3}y^{3}z^{3}=n} · This is a sum of cubes This is a semiimportant identity to know (x3 y3) = (x y)(x2 −xy y2) Although it doesn't apply directly to this question, it's also important to know that (x3 − y3) = (x −y)(x2 xy y2)Question дz Given the equation f(x,y,z)= x3 y3 z3 6xyz1, then will be equal to дх OA 2x2 2yz) O B None of the choices in this list OC x2 2yz OD X22yz OE x2 2yz 22 2xy 22 2xy 22 2xy 22 2xy This problem has been solved!




Rd Sharma Solutions For Class 8 Chapter 6 Algebraic Expressions And Identities Download Free Pdf




X Y 3 Y Z 3 Z X 3 3 X Y Y Z Z X 2 X3 Y3 Z3 3xyz Mathematics Topperlearning Com T86qex55
(1) "y3" was replaced by "y^3" 1 more similar replacement(s) Step 1 Trying to factor as a Sum of Cubes 11 Factoring x 3 y 3 Theory A sum of two perfect cubes, a 3 b 3 can be factored into (ab) • (a 2abb 2) Proof (ab) • (a 2abb 2) = a 3a 2 b ab 2 ba 2b 2 a b 3 = a 3 (a 2 bba 2)(ab 2b 2 a) b 3 = a 3 0= x^3y^3 z^3 3 x^2 y 3 x y^2 3(xy)^2 z 3(xy) z^2 =X^3 Y^3 Z^3 3 X^2Y 3XY^2 3 ( X^2 Y^2 2XY ) Z 3X Z^2 3YZ^2 =X^3Y^3Z^3 3X^2 Y3XY^2 3X^2 Z 3Y^2 Z 6XYZ 3XZ^2 3 YZ^2For a, b2Z, ab ab(mod ˇ), so the natural map Z=(3) !R=(ˇ) is a surjection of elds, hence an isomorphism Now it is more than enough to show that the equation x3 y3 = u(ˇkz)3 (12) has no solution in Rwith ua unit, k 1;x, y, and zpairwise coprime and xyznot divisible by ˇ We will show that (i) if there is a solution, then k 2;



Show That X3 Y3 Z3 3xyz X Y Z X2 Y2 Chegg Com
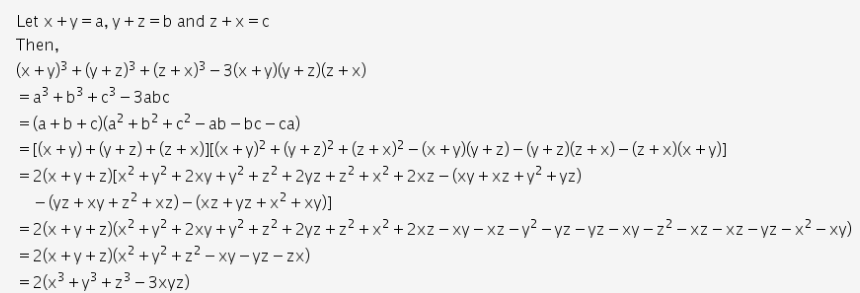



X Y 3 Y Z 3 Z X 3 3 X Y Y Z Z X 2 X3 Y3 Z3 3xyz Cbse Class 9 Maths Learn Cbse Forum
To ask Unlimited Maths doubts download Doubtnut from https//googl/9WZjCW Verify that `x^3y^3z^33x y z=1/2(xyz)(xy)^2(yz)^2(zx)^2`Factorize x^3 y^3 z^3 = 3xyz · The formula of x 3 y 3 z 3 – 3xyz is written as \(x^{3} y^{3} z^{3} – 3xyz = (x y z) (x^{2} y^{2} z^{2} – xy – yz – zx)\) Let us prove the equation by putting the values of x = 1;



Prove X3 Y3 Z3 3xyz Or Algebric Formula Dream Of Life Youtube




Solve For Integers X Y Z X Y 1 Z X 3 Y 3 1 Z 2 Please Also Suggest The Chapters From Where I Can Prepare For The Kinds Of Questions Mathematics Topperlearning Com 6lv499
Complete cubic parametrization of the Fermat cubic surface w 3 x 3 y 3 z 3 = 0 This is a famous Diophantine problem, to which Dickson's History of the Theory of Numbers, Vol II devotes many pages It is usually phrased as w 3 x 3 y 3 =z 3 or w 3 x 3 =y 3 z 3, with the implication that the variables are to be positive, as in the integer solutions 3 3 4 3 5 3 =6 3 (an amusing(((x 3)•(yz))y 3 •(zx))z 3 •(xy) Step 3 Equation at the end of step 3 (x 3 •(yz)y 3 •(zx))z 3 •(xy) Step 4 Trying to factor by pulling out 41 Factoring x 3 yx 3 zxy 3 xz 3 y 3 zyz 3 Thoughtfully split the expression at hand into groups, each group having two terms Group 1 y 3 zxy 3 Group 2 x 3 yx 3 zFirst type the equation 2x3=15 Then type the @ symbol Then type x=6 Try it now 2x3=15 @ x=6 Clickable Demo Try entering 2x3=15 @ x=6 into the text box After you enter the expression, Algebra Calculator will plug x=6 in for the equation 2x3=15 2(6)3 = 15 The calculator prints "True" to let you know that the answer is right More Examples



If 2 Power X 3 Power Y 6 Power Z Show That Z Xy X Y Mathematics Topperlearning Com 7984




If X A B Y B C Z C A Then X3 Y3 Z3 3xyz Brainly In
X^3 x y^3 y = z^3 z (1) where x, y, z are integers greater than 1 If z and x are both odd or both even, we can define integers u and v such that z=uv and x=uv Substituting into equation



Misc 16 Solve Equations 2 X 3 Y 10 Z 4 4 X 6 Y 5 Z 1




X3 Y3 Z3 Novocom Top



Maths Identity 8 Polynomial Part 12 English Youtube




Mathematics Class 9th Chapter 4 Solution




What Is The Distance Of The Point Math 3 4 5 Math From The Plane Math X Y Z 2 Math Measured Parallel To The Line Math 2x Y Z Math Quora




If X Y Z 0 Show That X 3 Y 3 Z 3 3xyz With Video




Simplify X 2 Y 2 3 Y 2 Z 2 3 Z 2 X 2 3 X Y 3 Y Z 3 Z X 3 Brainly In




Use Sympy To Do Math Programmer Sought




I Need An Example For This Formula X3 Y3 Z3 3xyz Brainly In




Complex Number Wikipedia




Contour Integration Wikipedia



Search Q X3 Y3 Formula Tbm Isch




Solving Simultaneous Equations The Substitution Method And The Addition Method Algebra Reference Electronics Textbook



If Y X 3 What Is The Value Of X Y Quora



How Do I Solve This System Of Equations Math Begin Cases Begin Bmatrix Begin Bmatrix 0 1 1 1 End Bmatrix 3 R 1 R End Bmatrix Frac Y X Frac Z Y Frac X Z 3 X Y Z 3 End Cases Math




Find The Value Of X Y And Z Calculator




4 Suppose X3 Y3 Z3 6xyz 1 Find A Chegg Com




Polynomials Ppt Video Online Download




Formula X Y Math Formulas
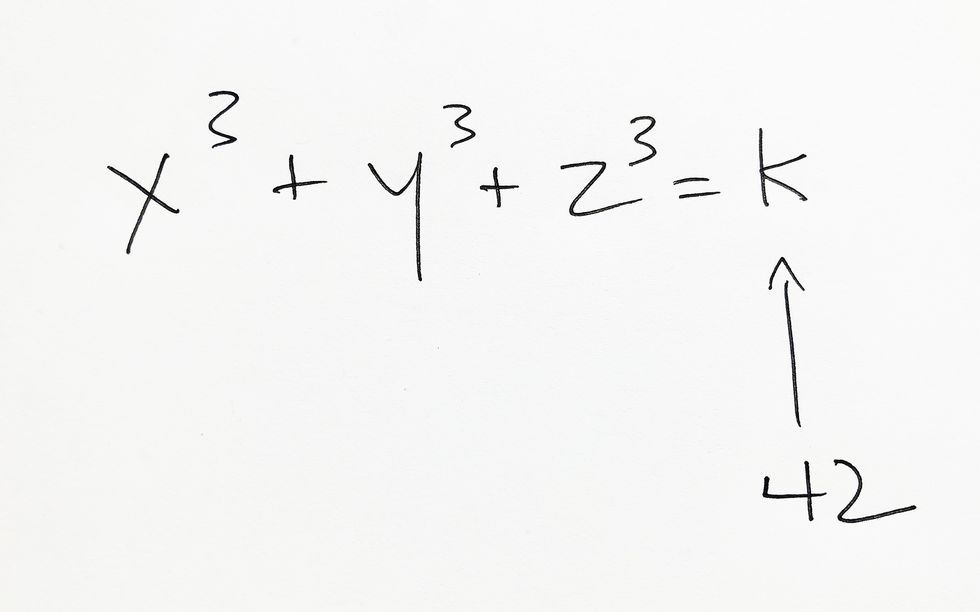



Hardest Math Problem Solved Diophantine Equation Answers



Pebsiones Si X 3 Y 2 Y Z 6 A Xy Z B X3 Gauthmath




Solve The Following System Of Equation By Matrix Method 1 X Y Z 6 X Y Z 4 X 2y 2z 1 2 X Y 3z 10 X Y Z 2 2x 3y 4z 4 3 X Y Z 6 X 2y 3z 6 X Y Z 2 4 2x Y Mathematics Topperlearning Com Yv1op566




Use Sympy To Do Math Programmer Sought



If Math X Y Z 0 Math How Do You Prove That Math X 3 Y 3 Z 3 3xyz Math Quora




If X Y Z 9 Then Find The Value Of 3 X 3 3 Y 3 3 Z 3 3 3 X 3 Y 3 Z Brainly In




Class Xii Practice Questions




How To Factorise Using The Identity X3 Y3 Z3 3xyz X Y Z X2 Y2 Z2 Xy Yz Zx Youtube
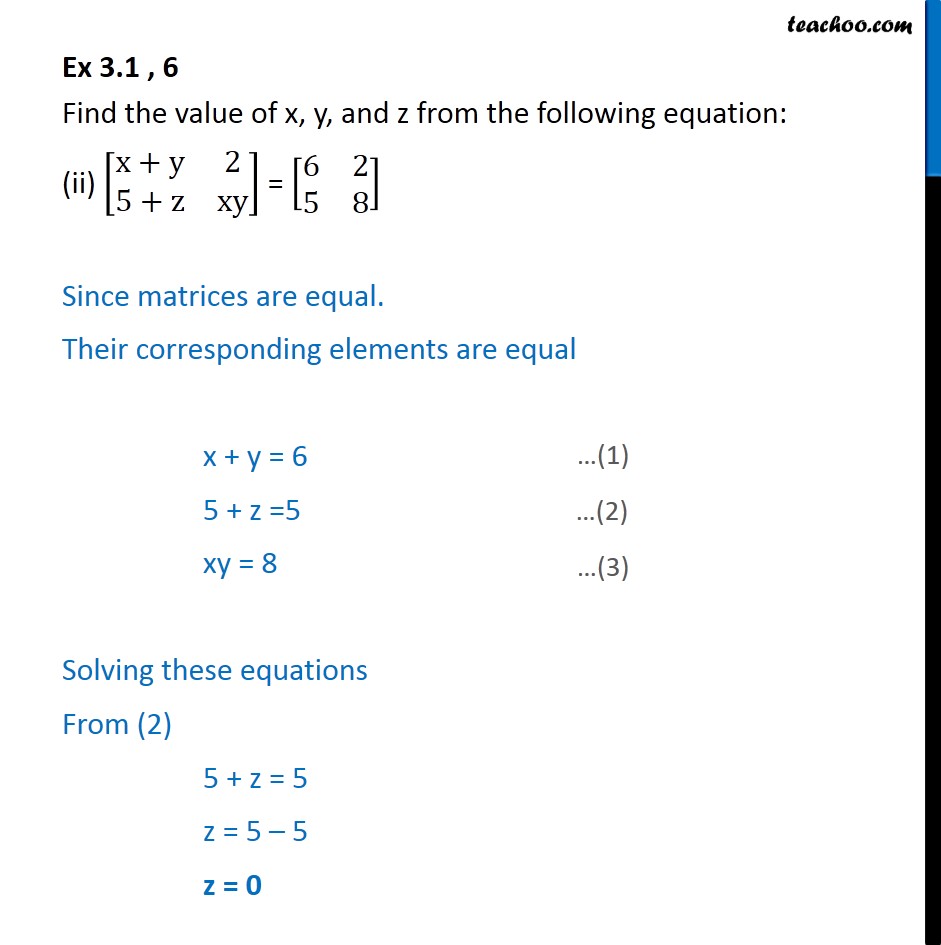



Ex 3 1 6 Find X Y Z From Equation Chapter 3 Matrices




Let R Be The Triangle With Vertices X1yi 2y2 Chegg Com




2 Two Cube 5 Five Cube 10 Ten Cube Pdf Free Download




If X A Y B Z C Then Show That X3 A3 Y3 Z3 A Xyz Abc Mathematics Topperlearning Com Mfmw0q55




What Is The Formula Of X3 Y3 Z3 Brainly In




The Points X 1 3 Y 8 3 And Z 2 1




If X Y Z 12 X 2 Y 2 Z 2 96 And 1 X 1




Ex 2 5 12 Verify That X3 Y3 Z3 3xyz 1 2 Ex 2 5
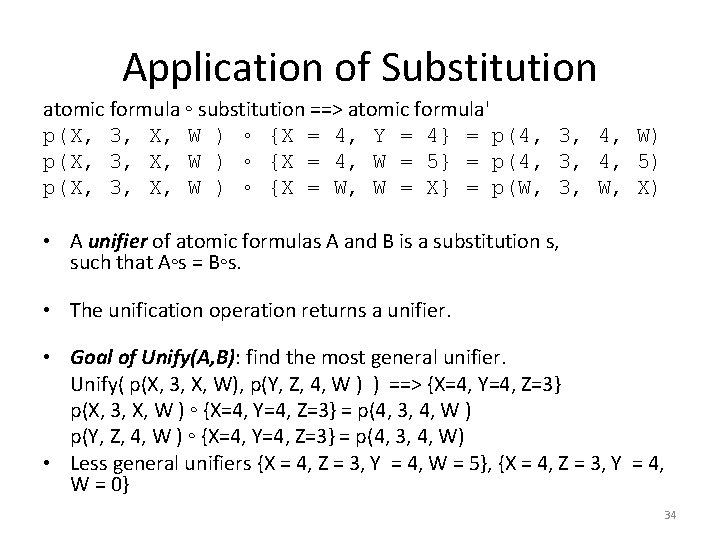



An Introduction To Logic Programming Chapter 6 Chapter




Prove That X Y 3 Y Z 3 Z X 3 3 X Y Y Z Z X 2 X3 Y3 Z3 3xyz Maths Meritnation Com
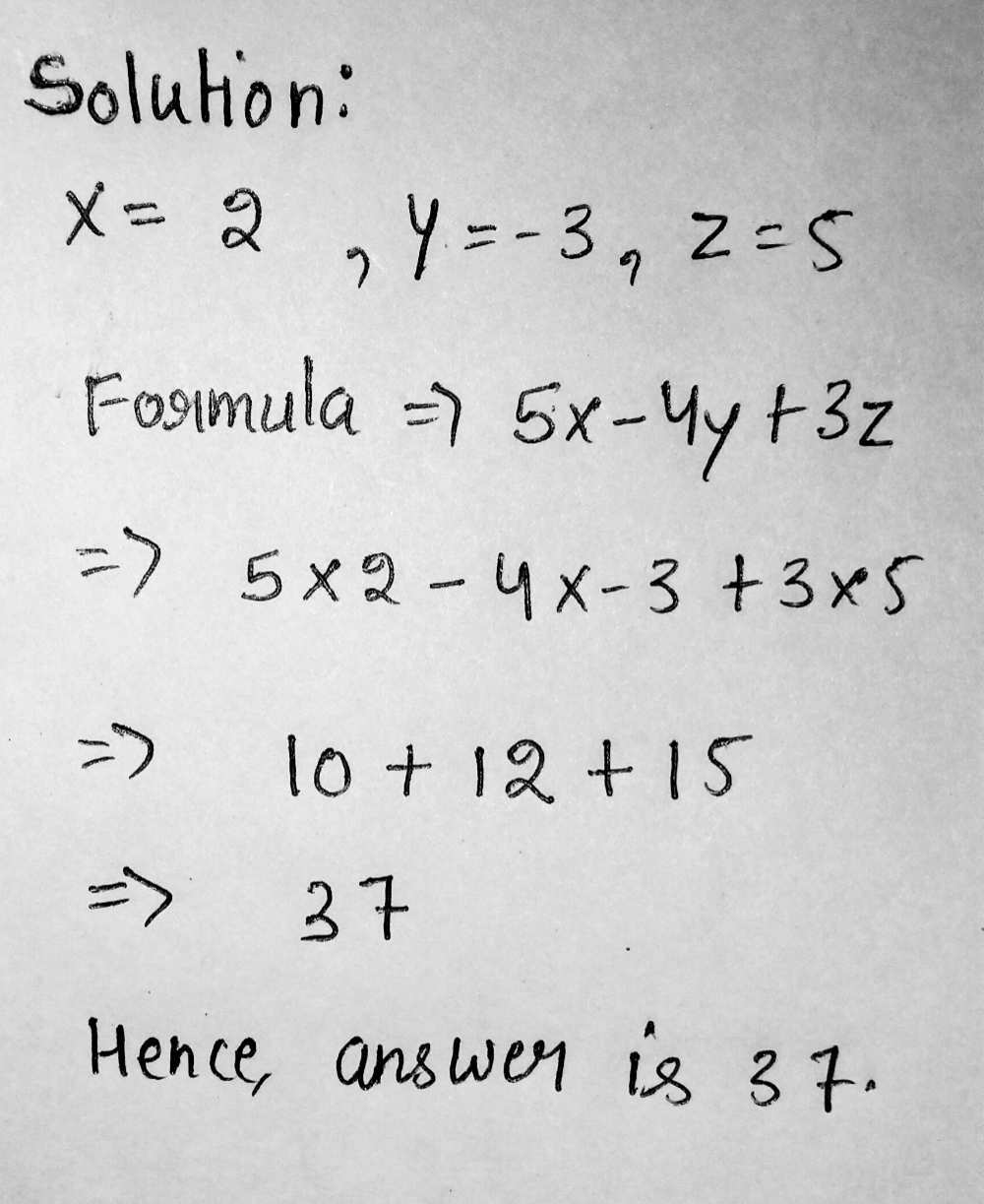



8ihelp86ogwq6m




X 3 Y 3 Z 3 3xyz X Y Z X 2 Y 2 Z 2 Xy Yz Zx A 3 B 3 C 3 3abc A B C A 2 B 2 C 2 Ab Ca Youtube




What Is The Formula Of Math A B 3 Math Quora




Verify X 3 Y 3 Z 3 3xyz 1 2 X Y Z X Y 2 Y Z 2 Z X 2 Brainly In




Prove That X Y 3 Y Z 3 Z X 3 3 X Y Y Z Z X 2 X 3 Y 3 Z 3 3xyz Youtube




Calc 501 1000 By James Bardo Issuu




X Y Z 3 4 5 And X 3 Y 3 Z 3 216 Then Find X Y Z Brainly In



Factorize Each Of The Following Expressions X 2 Y Z 3 3 X 3 2y 3 Y 3 5x 6 Y 3 4z 3 3 Sarthaks Econnect Largest Online Education Community




X3 Y3 Z3 Novocom Top




Formula
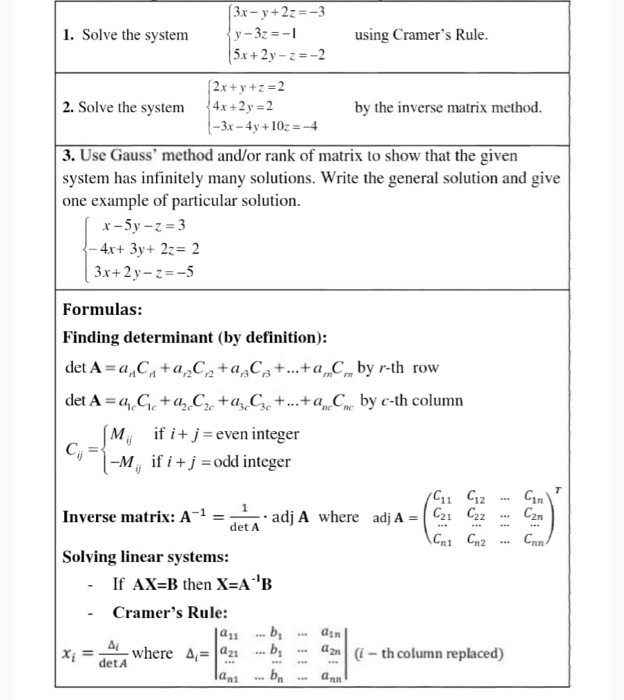



1 Solve The System 3x Y 2z 3 Y 3z 5x 2y 2 Chegg Com




Suppose A Temperature Function Is Given In R By The Chegg Com




Prove That X Y3 Y Z3 Z X3 3x Yy Zz X 2x3 Y3 Z3 3xyz Maths Polynomials Meritnation Com




Epa1 Elliptic Curve Encrypting Device Elliptic Curve Encrypting Method Elliptic Curve Encrypting Program And Computer Readable Recording Medium Recording That Program Google Patents




Prove That X Y Y Z Z X 3 X Y Y Z Z Y 2 X Y Z 3xyz Brainly In




Exercise 1 2 Cramer S Rule Problem Questions With Answer Solution




If X Y Z 9 Then Find 3 X Cube 3 Y Cube 3 Z Cube 3 3 X 3 Y 3 Z Brainly In




Today S Class Derivatives Of Multivariate Functions Ppt Download




Qos 3 If X Y Z 8 And X Y Y Z Z X Find The Value Of N X 3 Y 3 Z 3 3 X Y Z



1




X 3 Y 3 3 Y 3 Z 3 3 Z 3 X 3 3 X Y 3 Y Z 3 Z X 3 Youtube



How To Solve The System X 1 2 Y 1 2 27xy And X 2 1 Y 2 1 10xy Quora




Mathematics Class 9th Chapter 4 Solution



1
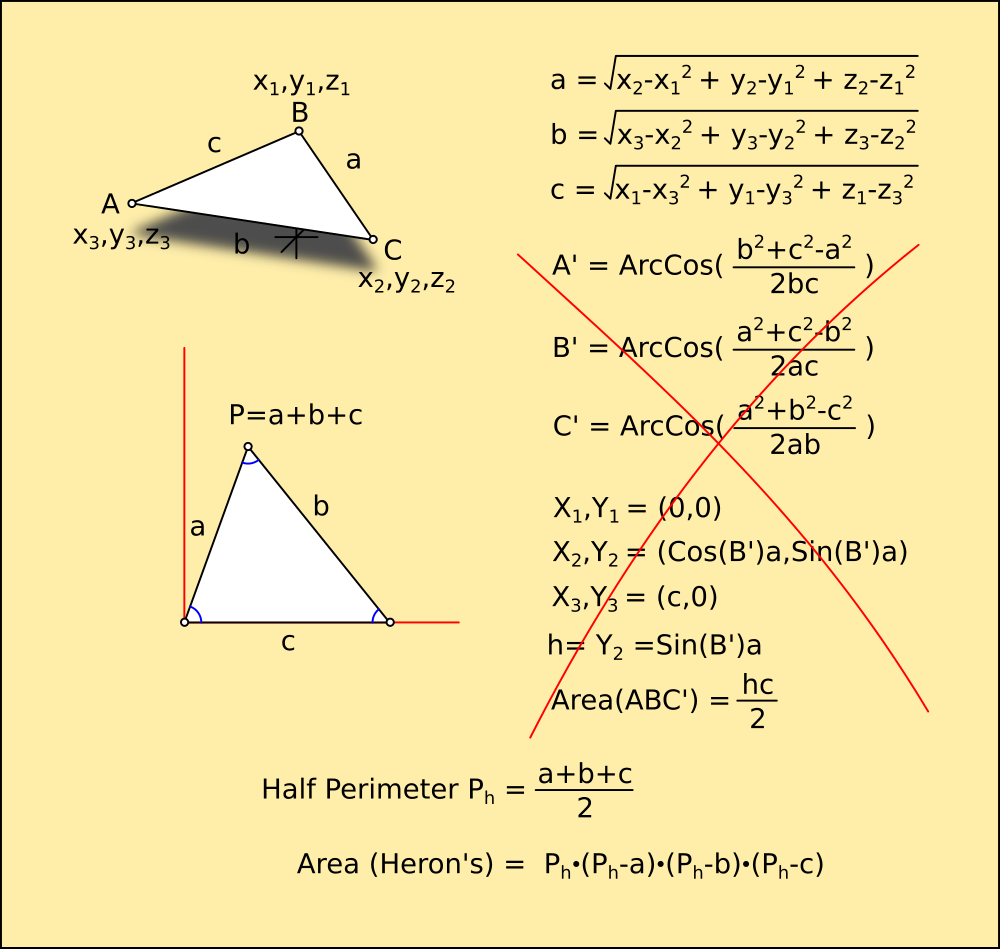



The Eternal Battle Light Vs Dark Details Hackaday Io




If X Y Z 0 Then Find The Value Of X3 Y3 Z3 Brainly In




If X Y Z 0 Then Show That X Cube Y Cube Z Cube 3xyz Mathematics Topperlearning Com Vj2f0044




Solved If X Y Z 1 Xy Yz Zx 1 And Xyz 1 Find The Value Of X 3 Y 3 Z 3 Brainly In




Verify That X3 Y3 Z3 3xyz 1 2 X Y Z X Y 2 Y Z 2 Z X 2 Class 9th Ex 2 5 Question 12 Youtube



Royalsocietypublishing Org Doi Pdf 10 1098 Rstl 1859 0019



X 3 Y 3 Z 3 3xyz Formula Proof Malaydras




Evaluate X3 Y3 Z3 3xyz When X 2 Y 1 And Z 3 Brainly In




Notes Up To Ch7 Sec3




X 3 Y 3 Z 3 42 Novocom Top




If 2 X 3 Y 12 Z Then Prove That Xy Z X 2y Mathematics Topperlearning Com 8v1liiww




Table 3 From Constructive And Destructive Use Of Compilers In Elliptic Curve Cryptography Semantic Scholar
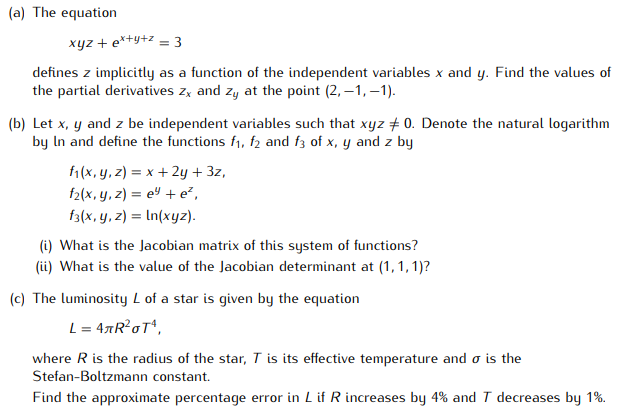



A The Equation Xyz E Y Z 3 Defines Z Chegg Com
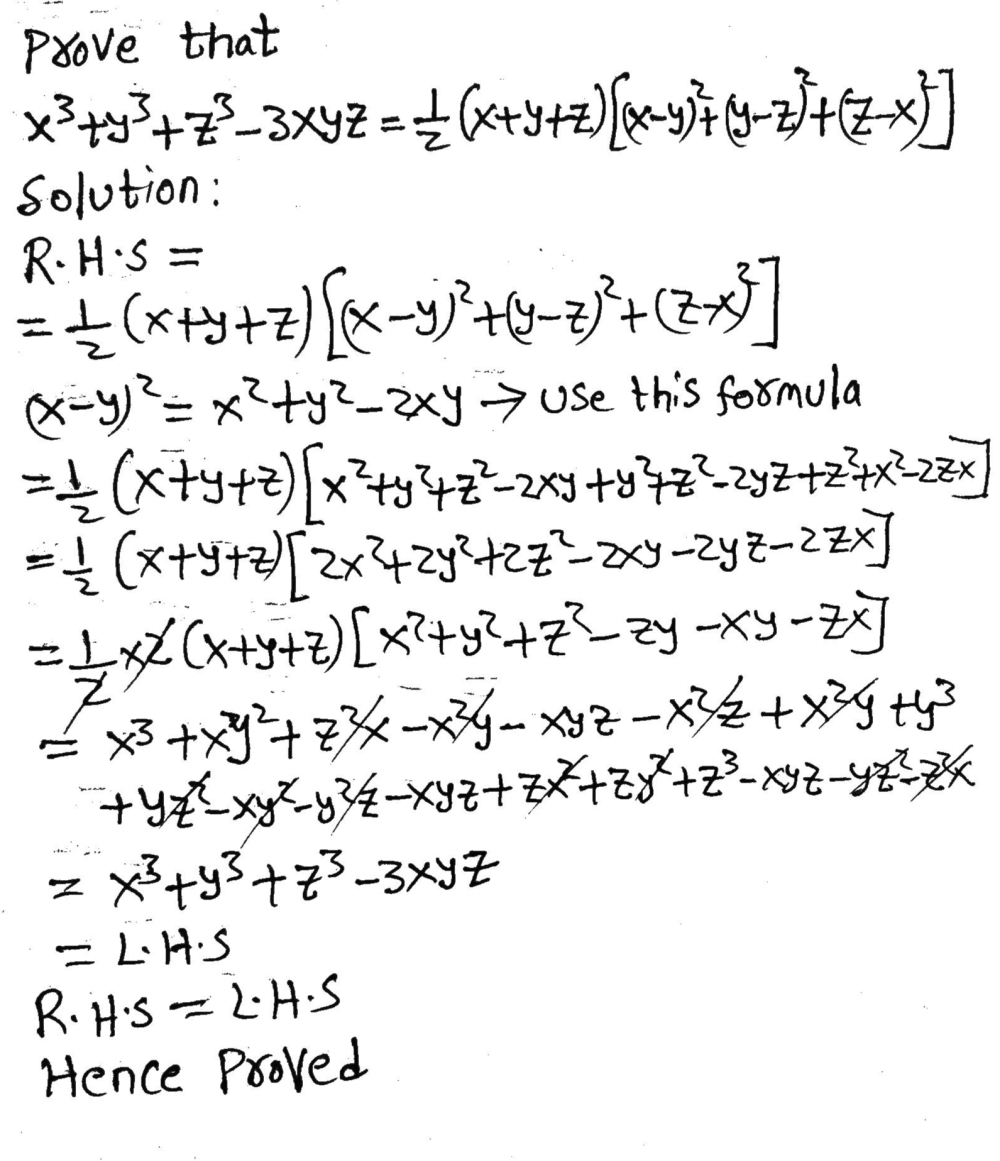



Verify That X 3 Y 3 Z 3 3xyz Frac 1 2 X Y Z X Y 2 Y Z 2 Z X 2 Snapsolve




The System Of Homogeneous Equations X Ky 2z 0 2x Y 3z 0 And 4x 2y Kz 0 Has Non Trivial Solution Then The Integral Value K Is




Bits Of Math Problem Cubes And Cube Roots



1


コメント
コメントを投稿